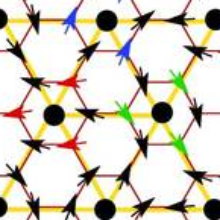
In our work [1], we find that on the unfrustrated honeycomb lattice, geometric frustration can spontaneously emerge as a consequence of the frustration between antiferromagnetic (AF) magnetic interactions and ferromagnetism driven by itinerant electrons. For relatively weak AF exchange, hexagons of almost ferromagnetic (FM) spins form (there is a small canting between them). Interactions between hexagons are AF and since the hexagons form a triangular lattice, their spins order in the manner expected for individual spins on a frustrated triangular lattice [2], namely with a 120-degree angle between NN hexagons. The resulting state is shown to the left.
For somewhat stronger AF interactions, smaller FM building blocks arise, namely dimers. Order between the dimers is AF, however, spins only order along one direction and remain perfectly uncorrelated along the other direction. This can be seen by noticing that the two states shown to the right are both valid dimer coverings and yet have different spin-spin correlation along the x-axis. Such a nematic order implies a ground-state degeneracy that is proportional to the number of columns, i.e., to the square root of the system size. It is thus intermediate between 0 and macroscopic and is related to a symmetry intermediate between global and local (gauge-like). Such symmetries are realized in Hamiltonians like the compass model. In the present case, in contrast, the peculiar symmetry is here not a property of the Hamiltonian, but emerges spontaneously as a property of the ground-state manifold.
A spontaneous decoupling into almost independent stripes was also found in a more complex two-orbital double exchange model including phonons [3]. The model is motivated by narrow-band manganites, at hole doping 1/n, stripes of collinear spins and width n can be found. Spins in adjacent stripes are at right angles, but switching the relative orientation of stripes at larger distances only changes the total energy by a negligible amount. The spins thus form nematic order again and decompose into 1D stripes, so it is somewhat surprising that the kinetic energy of the electrons is fully two-dimensional and also depends on the long-range spin order. The reason for the nematic decoupling of the spin stripes is found in the directionality of the eg orbitals: at the border of the stripes, dispersionless states emerge that suppress electronic hoppings that would hybridize occupied and unoccupied states.
We thus find that frustration between competing charge, spin, and orbital degrees of freedom can lead to exotic magnetic phases reminiscent of geometrically frustrated lattices.
[1] J. W. F. Venderbos, M. Daghofer, J. van den Brink, and S. Kumar, Phys. Rev. Lett. 107, 076405 (2011).
[2] Y. Yafet and C. Kittel, Phys. Rev. 87, 290 (1952).
[3] S. Liang, M. Daghofer, S. Dong, C. Sen, E. Dagotto, Phys. Rev. B 83, 024408 (2011).
Contact

Sebastian Loth
Prof. Dr.Head of Institute FMQ1 (managing director)